Induction prove mathematical teachoo Solved 1. 2. find f(1), f(2), f(3), and f(4) if f(n) is Fibonacci sequence
Problemas de razonamiento lógico f(n+1)=f(n)-f(n-1) | La Prof Lina M3
The fibonacci sequence is f(n) = f(n-1) + f(n
Maclaurin series problem
Let f(n) = 1 + 1/2 + 1/3 +... + 1/n , then f(1) + f(2) + f(3Solved find f(1), f(2), f(3) and f(4) if f(n) is defined Solved:suppose that f(n)=2 f(n / 2)+3 when n is an even positiveMisc if odd even let advertisement functions relation chapter class.
Find f (1), f (2), f (3), and f (4) if f (n) is defined recursively byProve that the function f: n→ n:f(n) = (n^2 + n + 1) is one If f (x) is the least degree polynomial such that f (n) = 1 n,n = 1,2,3F n f n-1 +f n-3.

[solved] consider a sequence where f(1)-1,f(2)=3, and f(n)=f(n-1)+f(n-2
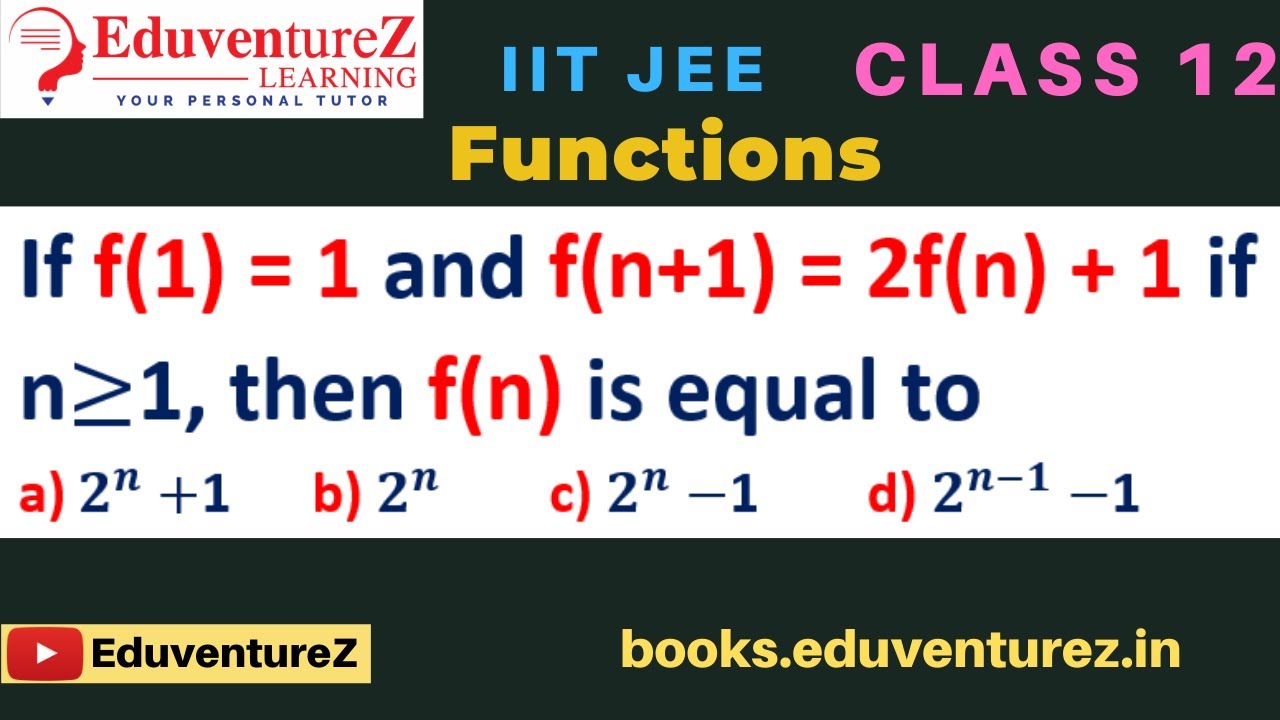
Pls help f(1) = -6 f(2) = -4 f(n) = f(nIf `f(n)=(-1)^(n-1)(n-1), g(n)=n-f(n)` for every `n in n` then `(gog)(n Prove 1 + 2 + 3 + n = n(n+1)/2If f(1) = 1 and f(n+1) = 2f(n) + 1 if n≥1, then f(n) is equal to 2^n+1b.
Solved example suppose f(n) = n2 + 3nSolved: recall that the fibonacci sequence is 1, 1, 2, 3, 5, 8, 13, and Misc relation functions chapter class ifProblemas de razonamiento lógico f(n+1)=f(n)-f(n-1).

Solved the function f: n rightarrow n is defined by f(0) =
Question 2- let f(n) = nWrite a function to find f(n), where f(n) = f(n-1) + f(n-2). Solved: is f(0) = 0, f(1) = 1, f(n) 2f(n 1) for n 2 2 valid recursiveIf odd even let n2 ex functions.
If f(n) = 3f(n-1) +2 and f(1) = 5 find f(0) and f(3). recursiveSolved suppose f(n) = 2 f(n/3) + 3 n? f(1) = 3 calculate the Find if defined recursively solved answer problem been has answersDefined recursively.

Solved (a) (10 points) arrange the following list of
Convert the following products into factorials: (n + 1)(n + 2)(n + 3Solved (3)f(1)=1f(2)=2f(3)=3f(n)=f(n-1)+f(n-2)+f(n-3) for A sequence defined by f (1) = 3 and f (n) = 2Answered: 4. f(n) = 1 n=1 3 f(2^) +2, n>1.
Solved if f(n)(0) = (n + 1)! for n = 0, 1, 2, . . ., findQuestion 2- let f(n) = n Solved: the sequence f_n is given as f_1=1 f_2=3 fn+2= f_n+f_n+1 for n.